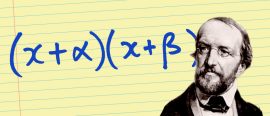Articles by Adam Townsend

Squid Game, hidden harmonies and DnD coming your way in our brand new issue! Plus all your favourite puzzles & columns.

Ellen talks to the mathematician and scientist about Attenborough, arctan and Antarctica

Win a £100 Maths Gear goody bag by solving our infamous puzzle

E Adrian Henle, Nick Gantzler, François-Xavier Coudert & Cory Simon team up for a deadly challenge

Weddings, holidays and catfish find their way into the prof's postbox this issue.

Goran Newsum always should be someone you really love

Poppy Azmi explores the patterns that are all around us

Can you solve it?

Mats Vermeeren sketches a simple proof of Noether's first theorem

Board yet? You will be.

Are you β? Are you γ?

Madeleine Hall looks at one of the minds behind GPS

The Chalkdust editors share some of their favourites

Chris Boucher explores the secrets and symmetries behind a measure of the distance between binary strings

We announce the winner of this coveted prize

The definitive chart of the best matrices

Have your say

Fashion is fleeting, Chalkdust regulars are not.

Julia Schanen hates being mistaken for a genius.

Callum Ilkiw takes us through a Dungeons and Dragons dice dilemma

How Kelvin estimated the age of the Earth by thinking about a baked potato

Sophie Bleau uncovers the secrets behind covering maps

Lucy Rycroft-Smith and Darren Macey unpick the legacy of some of the most ubiquitous names in statistics

Roll up, roll up! Squid Game, hidden harmonies and DnD coming your way in Issue 15. (Plus all the usual nonsense.)

Did you solve it?

We review the seventh of this year’s nominees for the Book of the Year

We review the sixth of this year’s nominees for the Book of the Year

We review the fifth of this year’s nominees for the Book of the Year

We review the fourth of this year’s nominees for the Book of the Year

We review the third of this year’s nominees for the Book of the Year

We review the second of this year's nominees for the Book of the Year

We review the first of this year's nominees for the Book of the Year

We announce the shortlist of our favourite maths-themed books of last year

Have your say, and help to choose the 2021 Readers' Choice

Venn diagrams, retrograde chess, and behind-the-scenes Christmas lectures all feature in our autumn 2021 edition. Plus all your favourite puzzles & columns.

Donovan Young interferes in wave patterns

Win a £100 Maths Gear goody bag by solving our infamous puzzle

Madeleine Hall takes a brief dive into the world’s favourite set-relationship-representation diagram.

Football, telly and shape-shifting houses find their way into the prof's postbox this issue.

Forgotten how to draw a log graph? No need to panic – here's a handy guide!

Ellen Jolley learns how to run a maths outreach programme

Can you solve it?

Michael Wendl dissects some variants of the magic separation, a self-working card trick.

How did your favourite sweater become so big? And how big can it get?

The definitive chart of the best waves

Paddy provides a much anticipated update to the most important use of statistical analysis in the last two years

Dimitrios Roxanas tells us why playing chess backwards is the new black (and white).

Tanmay Kulkarni intentionally gets lost on the Tokyo subway

Fashion is fleeting, Chalkdust regulars are not.

Kimi Chen deciphers the 1920s story you haven’t heard

Hollis Williams explores the power even simple models can have in describing the world around us.

The Leibniz–Lagrange–Newton showdown we all want to see

Have your say on the top ten matrices

Enter stage left: Chalkdust issue 14. Preorder now!

Did you solve it?

Did you solve it?

Sus Mafia strategies, LMS president Ulrike Tillmann, and Bae's theorem ❤️ all feature in our spring 2021 edition. Plus all your favourite puzzles & columns.

Sophie Maclean and David Sheard speak to a very top(olog)ical mathematician!

Madeleine Hall explores the sometimes counterintuitive consequences of conditional probability to our everyday lives.

Sophie explores the fascinating mathematics behind the games Mafia and Among Us.

Paddy Moore levels the score

Issue 13 is here! Find out how to get involved with our mathematical art launch project

We hear arguments for and against this controversial convention

Francisco Berkemeier takes a look at the mathematics behind elections, and the Electoral College, in the United States of America.

Have your say

Discover the meaning of the coloured squares on the cover of issue 13

Fashion is fleeting, Chalkdust regulars are not.

Are you binary? Roman numerals? The decimals? Find out by answering a maximum of ∞ easy questions

The story of an unforgettable mathematician.

£100 of Maths Gear goodies to be won if you can solve it

Parishoners, partners and puzzled playwrights have written in to the professor's postbox this issue

Johannes Huber explores the maths behind how image compression works.

Aryan Ghobadi gives a maths lecture at a zoo

How big is the biggest cow?

The definitive chart of the best buttons

Looking for a challenge? Try our cryptic crossnumber—with hints and tips so anyone can have a go!

A great start to the month of Maying

We announce the winner of this coveted prize

Have your say, and help to choose the 2020 Readers' Choice

We review the seventh of this year's nominees for the Book of the Year, and open the vote for the readers' favourite

We review the sixth of this year's nominees for the Book of the Year

We review the fifth of this year's nominees for the Book of the Year

We review the fourth of this year's nominees for the Book of the Year

We review the third of this year's nominees for the Book of the Year

We review the second of this year's nominees for the Book of the Year

We review the first of this year's nominees for the Book of the Year

We announce the shortlist

Łukasz takes us on a tour through a surprisingly diverse range of algorithms to test for divisibility by 7

We reveal the solutions to our Christmas puzzles!

Puzzle #3 in our 2020 Christmas puzzle series

Puzzle #2 in our 2020 Christmas puzzle series

Puzzle #1 in our 2020 Christmas puzzle series

Belgin gets hooked on a classic maths game...in 16 bits! Here's her review...

Try out these flo-maps for yourself: fractions speak louder than words

Hugh Duncan returns with the long-awaited prequel in which he further explores the geometric patterns hidden behind the fractions.

Now available to catch up on YouTube

Independent Sage's Christina Pagel, hyperbolic mindfulness and make-your-own Markov tweet feature in our autumn 2020 edition. Plus all your favourite puzzles & columns.

Ellen Jolley asks for (independent) Sage advice

Books, business and barns are the topics readers have sent in to the professor's postbox this issue

£100 of MathsGear goodies to be won if you can solve it

Maynard manages to prove that 2≠1 in less space than it took Bertrand Russell to prove that 1+1=2

Florian Bouyer explains the beautiful geometry behind his mathematical colouring-in designs.

A handy tool that can make writing great tweets much easier

Who is behind the so-called Nobel prize of mathematics? Gerda Grase investigates.

Sam Hartburn attempts the impossible

Does maths need a Nobel prize?

Emilio McAllister Fognini explores the maths that made Turing so famous

Fashion is fleeting, Chalkdust regulars are not.

Make your own scatterer from some old Christmas decorations

I love Markov is I love Markov chains love me.

Scroggs debates whether sharing truly is caring

Solitons

Have your say

Colin counts Countdown's contingent of conundrum causing calculations

James M Christian reflects on chaos

The definitive chart of the best day trips

Did you solve it?

Adam Townsend sets the fifth and final puzzle. Can you solve it?

Humbug sets the fourth puzzle. Can you solve it?

David Sheard sets the third puzzle. Can you solve it?

TD Dang sets the second puzzle. Can you solve it?

Space-filling curves, cheating at cards and automated joke generation feature in our spring 2020 edition. Plus all your favourite puzzles & columns.

Celebrate the launch of issue 11 by taking part in our puzzle hunt

We chat with Trachette about her work in mathematical oncology, her role models, and boosting diversity in mathematics

£100 of MathsGear goodies to be won if you can solve it

Are you a torus? A cone? Ed Spheran? Find out by answering a maximum of five easy questions

Kevin Houston teaches us how to deal ourselves the best hand

Grant applications, musical media and last-minute Olympics training are the topics readers have sent in to the professor's postbox this issue

Mara Kortenkamp, Erin Henning and Anna Maria Hartkopf give us a tour of Polytopia, a home for peculiar polytopes

I like my towns like I like my Alex: Bolton

Sam Hartburn orders wine by the barrel, but wonders if she's getting the most wine

Nobody could draw a space filling curve by hand, but that doesn’t stop Andrew Stacey

Vote for your favourite maths-themed day out

Some saucy modelling

Like Fibonacci, but weird. Robert J Low and Thierry Platini explain

Make your own Ecki

David Sheard explores the rich mathematics and history behind the Apollonian packing, and the cover of issue 11

Fashion is fleeting, Chalkdust regulars are not.

The definitive chart of the best pictures of scorpions

Yuliya Nesterova orders some polynomials around

Matthew Scroggs sets the first puzzle. Can you solve it?

Issue 11 of everyone's favourite magazine for the mathematically curious is coming very soon

Did you solve it?

Issah Merchant discusses the geometric principles behind, and real-world applications of, curvature

We announce the winner of this coveted prize

Have your say, and help to choose the 2019 Readers' Choice

We review the ninth of this year's nominees for the Book of the Year

We review the eighth of this year's nominees for the Book of the Year

We review the sevnth of this year's nominees for the Book of the Year

We review the sixth of this year's nominees for the Book of the Year

We review the fifth of this year's nominees for the Book of the Year

We review the fourth of this year's nominees for the Book of the Year

We review the third of this year's nominees for the Book of the Year

We review the second of this year's nominees for the Book of the Year

We review the first of this year's nominees for the Book of the Year

We reveal the shortlist

21 simple steps to draw your own Islamic pattern!

Computational proofs, AI music and embarrassing surveys feature in the autumn 2019 issue. Plus all your favourite puzzles & columns.

Can you solve it?

And will we soon all be out of a job? Kevin Buzzard worries us all.

Letter writing, hospital visits, and getting the family active are among the topics of discussion in this issue's Dear Dirichlet advice column

We chat to the crypto chief about inventing RSA... but not being able to tell anyone

Part 7 of our mathematical comic's adventure

Yiannis Petridis connects square roots and continued fractions

Pamela E Harris's story, as told by Talithia Williams

Carmen Cabrera Arnau explores the use of AI in composition

How do you like your numbers? Prime? Positive but infinitely small? Find out which famous mathematician you are

Angela Brett might not be standing on their shoulders

This article will (unsurprisingly) tell you how to crochet a fractal

Ever thought about making your own fractal?

Explaining the mathematics of tiling, and the cover of Issue 10

Fashion is fleeting, Chalkdust regulars are not.

The definitive chart of the best issues of Chalkdust

You won't bee-lieve it

Paula Rowińska uses mathematics to answer some awkward questions

Vote for your favourite picture of a scorpion

Andrei Chekmasov explores order and infinity

Tasty and mathematical

Come along to our launch party for free pizza and the real quiz

A month celebrating the contributions of black mathematicians

Did you solve it?

Hugh Duncan explores an exciting variation on Conway's Game of Life

We have a go at the puzzles in Daniel Griller’s new book

W.L. Feldhusen explains the obscure sine-finding trick hiding inside your calculator!

Tony Pisculli dissects the lyrics from a popular song

The scientific story behind the cover of Joy Division's treasured debut

A podcast for the mathematically curious

In 2018, scientists discovered a new shape that is essential to multicellular life

Ride a phantom parabola into our spring 2019 issue. Billiards, maths, tiles, mistakes, plus all your favourite regulars.

Win £100 of Maths Gear goodies by solving our famously fiendish crossnumber

Stephen Muirhead meets neither, as he explores waves, tiles and percolation theory

Coffee, Brexit and badgers are among the topics of discussion in this issue's Dear Dirichlet advice column

Yuliya Nesterova misses all the pockets, but does manage to solve some cubics

Mystic mug has some predictions for you...

Axel Kerbec gets locked out while exchanging keys

The definitive chart of the best Chalkdust regulars

Interviewing Matt was a mistake

Lucy Rycroft-Smith reflects on the use of this well-established measurement

Your guide to creating the optimal homepage

Find out more about the spiral trees on the cover of Issue 09

Fashion is fleeting, Chalkdust regulars are not.

Are you u or i? Am I? Who?

An adventure that starts with a morning of bell ringing and ends with a mad dash in a taxi

Vote for your favourite Chalkdust issue

Zoe Griffiths investigates paranormal quadratics

Using graph theory to predict who will sit the iron throne

Peter Rowlett uses combinatorics to generate caterpillars

How big are these random shapes? Submit an answer for a chance to win a prize!

Celebrate International Women's day by reading about rebel women in mathematics!

More sartorial inquisition for your feet

Read the magazine, and come to the launch party!

A podcast for the mathematically curious

Did the Danish mathematician also sail the high seas?

Did you solve it?

Tricks and puzzles that provide an introduction to the world of partitions

A podcast for the mathematically curious

How can we teach people about the sieve in a way that helps them best understand prime numbers?

Investigating the power of thinking rationally

Looking back at puzzles about complex numbers, tic-tac-toe, and the Eggnog Mystery

Lies, liquor and logical deduction play their part in this festive holiday tale

Why do Christmas lights get tangled? And what's the perfect way to decorate a Christmas tree? Find the answers here.

Win this year's best book of geometry puzzles

Looking back at the exciting day that closed our 2018 celebrations

Win a DVD boxset from Festival of the spoken nerd in our first Christmas competition!

Mike Fletcher explores the optimal strategy for winning the popular television game show

Bring your shovel, and dig with us to unearth some amazing results about polynomials

A podcast for the mathematically curious

Hugh Duncan explores polygons with a shortage of edges

What is pi? How do we define it and who first thought of it? We explore the history of this quintessential mathematical constant.

Introducing Mathscon, a mathematics conference with a difference!

John Pougué Biyong explains how and why science communication can lead to better diversity.

Reviewing the Royal Institution's first Black History Month event.

Surprise yourself by making this thing

Swing on a magnetic pendulum into our autumn 2018 issue. Topological tic-tac-toe, maths, cake, categories, plus all your favourite regulars.

Print it out and play against your friends!

We chat to the author of the best-selling book How to Bake Pi and pioneer of maths on YouTube

Win £100 of Maths Gear goodies by solving our famously fiendish crossnumber

Colin Beveridge looks at different designs for 2- and 3-dimensional tiles

Alex Bolton plays noughts and crosses on unusual surfaces

Read about Maxamillion Polignac's adventures in a prime-hating world

Adam Atkinson uses maths to try to help a sculptor

See what we're doing this October to promote and celebrate diversity in mathematics

Are you perfect or imaginary? Are you a square?

Find out more about the weird shapes on the cover of Issue 08

Village fetes, Brexit and flowers are among the topics of discussion in this issue's Dear Dirichlet advice column

Elizabeth A Williams falls off a log

The definitive chart of the best units

Emma Bell explains why the Renaissance mathematician Gerolamo Cardano styled himself as the "man of discoveries".

Tired of hearing people ask which area of maths is your favourite? Get one of these tattooed on your forehead and you'll never be asked again...

Vote for your favourite Chalkdust regular

Many mysteries about hair are now revealed with the ponytail shape equation

Fashion is fleeting, Chalkdust regulars are not.

Just what is category theory? Tai-Danae Bradley explains

Biography of Katherine Johnson, NASA human computer and research mathematician

A tabletop demonstration of chaos.

Nira Chamberlain explains how Black Panther's suit can be modelled mathematically

Clive Fraser reflects on his interactions with one of the greatest ever Black mathematicians

This month’s round up of mathematical blog posts from all over the internet

We look back at last year's Black Mathematician Month, and give a preview of what to expect this October.

You'll never believe number 3!

Join us at our upcoming launch party!

We explore the concept of emptiness in set theory, and explain how zero went from "nothing" to "something"

Introducing the work that has won mathematics' most famous award

Exploring the beauty of complex numbers, their origins and why they are important

How does one produce a net for the broadest class of polyhedra?

... and how Chalkdust played a role in one of them

Did you solve it?

Exploring non-random walks using fractions

A review of Vicky Neale's new book about the quest to understand prime numbers.

We analyse the maths and physics required to execute a good chip shot

Discover the mathematical equations that describe the most commonly observed trajectories in football

An unexpected way to beat the odds in this classic game

Defining what exactly an integral is leads naturally to an explanation of how to handle approximating them.

A selection of weird goings-on from the world of fluid mechanics

A thrilling review of this truly enlightening book

Blood is exceptionally complicated and its composition varies from person to person. So how do we begin to model it?

A mathematically-themed version of the classic card game, with several new features

A collection of our favourite and least favourite things named after Euler, from issue 07

Have you been wondering what the pattern on it means?

``Read Euler, read Euler, he is the master of us all." -- Laplace. An invitation to join us in celebrating Euler's 311th birthday by appreciating a few of his great contributions to mathematics.

Or, how a simple problem can get very complicated, very quickly...

Let's take a look at patterns that can be discovered in Fibonacci numbers and how we can find them around us.

Think outside outside the box in our spring 2018 issue. No more tennis puns, primes mod 4, plus all your favourite regulars.

No more Katie Steckles.

Rob Eastaway joins the dots.

Pigs, popes and produce are among the topics of discussion in this issue's Dear Dirichlet advice column

Win £100 of Maths Gear goodies by solving our famously fiendish crossnumber

Sam Hartburn bakes your favourite fractal

Zoe Griffiths on the life of e

High stakes gambling with Paula Rowińska

Part 6 of our mathematical comic's adventure

In this edition of the series, we instead learn about 'routes' and Edsger Dijkstra's shortest path algorithm.

Alex Xela shows us the world of palindromic numbers, and calculates the chances of getting one

Find out more about the patterns on the cover of Issue 07

The definitive chart of the best dates

Biography of Sir Christopher Zeeman

Race to be the first to get five-in-a-row

Infinitely many primes ending in 1, 3, 7 and 9 proved in typically Eulerian style.

Vote for your favourite unit

Make a catastrophe machine just like Zeeman's

Start your quest to conquer the planet with this introduction to the wonderful world of machine learning

Modelling unemployment using simple differential equations!

Undoubtedly the most influential voice on this hottest of hot topics.

John Dore and Chris Woodcock join the dots

We chat to one of the UK's most qualified voices in mathematics communication

Fashion is fleeting, Chalkdust regulars are not.

You won't believe number 5!

What is the probability that d+2 random points in d-dimensional space form a convex body? Investigating an old problem using modern methods.

Join us at our upcoming launch party!

"Unlocking the hidden mathematics in video games"

Learn how to model your heart one beat at a time ❤️

Belgin plays a classic mathsy game from her childhood...in 16-bit graphics! Here's her review...

You can un-knot a knot, by cutting it not?

Why do surnames die out? We take a look at the Galton-Watson process for modelling the extinction of surnames to answer the question: 'When will we all be Smiths?'

Did you solve it?

A fiendish puzzle for you to 'pour' over...

Some summations seem strangely slippery...

Uniting the teaching of mathematics and music can benefit pupils greatly in both areas.

What have we been up to this year?

... and the winners

Can you solve four puzzles to reveal the hidden message?

Reviewing this week's conundrum prize... science-y stuff staring you in the face!

Try our nonograms! And remember, not all puzzles have a unique solution...

Christmas is coming, and Santa will soon begin his journey. We analyse the science and maths behind his trip.

Solve this and you could be the lucky winner of a signed copy of The Indisputable Existence of Santa Claus

We round up some of the last month's top mathematical posts from around the internet

Solve this and you could be the lucky winner of a Chalkdust T-shirt

As the festive season strikes again, we'll be dishing out more fiendish puzzles – this time with prizes!

Should you ask Santa for 'Ice Col' Beveridge's encyclopedic tome this festive period?

Chalkdust descends upon the UK's largest pop maths gathering and tells you what you missed

When you get tired of using your calculator for numbers, why not use it for words?

Maths has strong connections to art and music, but what about to both at the same time?

Reflecting on what we've learnt over the past few weeks.

The co-author of a recent paper on diversity in professional STEM societies talks about access to science.

Meet Talitha Washington, an activist, mathematician, and professor

Stories and lessons on diversity in mathematics from a globe-trotting number theorist.

We spoke with Jonathan Farley about his research and experiences as a black mathematician.

As part of Black Mathematician Month, we spoke to the Bristol University professor about access schemes and the importance of mentors.

Cardioids in coffee cups, counting with three fingers, and breakfast at Villani's. Plus the return of all your favourite fun pages in our autumn 2017 issue.

Win £100 of Maths Gear goodies by solving our famously fiendish crossnumber

We feel underdressed for Breakfast at Villani's

Prof. Dirichlet tackles archaeology and supercomputers in answering your personal problems

Staring at your coffee, you wonder whether the light reflecting in cup really is a cardioid curve...

Robert J Low flips one upside down.

Part 5 of our mathematical comic's adventure

We take a proper look at her mathematical accomplishments

"R" you a Matlab or a Python? Fortran or Mathematica? Find out which package suits you best.

The definitive chart of the best tools

A biography of Sophie Bryant

Mathematical fashion advice for an ever-changing world

Vote for your favourite mathematical celebration day

Murder, maths, malaria and mammals

Blaise Pascal was driven to begin the mechanisation of mathematics by his father's struggles with an accounts book in 17th century France.

Euclidean Egg III makes the cover of issue 06!

Contemplate the beauty of the Julia and Mandelbrot sets and an elegant mathematical explanation of them

Modelling a Saturday afternoon on Oxford Street

A great way to waste a lot of paper

20 questions, the axiom of choice and colouring sequences.

How many did you spot?

Stories that illustrate the barriers that black mathematicians have faced in recent history.

Data scientist Jonny Brooks-Bartlett gives his views as part of Black Mathematician Month.

Meet Olubunmi Abidemi Fadipe-Joseph, an active promoter for women in mathematics from Nigeria

Nira Chamberlain, one of the UK's top 100 scientists, shares his experiences as a black mathematician.

Natalya Silcott introduces us to star polynomials!

Our first interview celebrating Black Mathematician Month

Promoting black mathematicians, and talking about building a more representative mathematical community.

Free launch party, 7.15pm. Come along!

A polygon with four and a half sides?!

While drinking beer in your favourite pub, have you ever wondered how it is produced? Find here some of the science and mathematics behind brewing.

Take a ball, divide it into parts, glue them back and get two identical copies of your ball!

A hideous equation that hides beautiful images, and much (much) more besides...

How processes used in image editing are related to mathematics!

We created hot ice from scratch, a solution that remains liquid even below its freezing point!

A quick look at how to get the most bang for your buck the next time you're in a bidding war

A summer essential or an embarrassment risk on the streets of Ibiza?

How to win a game when your expected score is 0

Did you win?

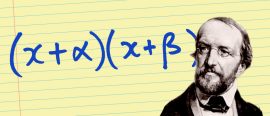
Write down a quadratic. What is the probability that it factorises? Paging Prof. Dirichet...

A crossnumber clue takes us plunging headfirst towards projective geometry

Human migration with mathematical models, data and a hands-on experiment!

Some interesting observations with the pigeonhole principle

Have a go at these puzzles, adequate for your holidays!

We take a look at the top 10 emojis!

Next time you are finished using a paper cup, create your own papercupter!

Read about our least favourite numbers in this collection from issue 5!

Discover the mysteries behind the most spectacular free-kick ever scored and how Newton can help us to simulate it.

Finding the best picture at the Leaning Tower of Pisa is all about the maths rather than the pose!

Each time you eat a croissant you might be biting more than 500 layers of dough!

We have a go at the puzzles in Daniel Griller's new book

We chat to Marcus about science communication, teaching mathematics in schools, and how to make group theory sound sexy.

Negative polygons and other mathematical creations

In the Aztec city of Atzlan, the scientist Remotep makes a revolutionary discovery

How optimising the space around a circular monument is related to supereggs

When you're caught in the rain without an umbrella... what is your best option?

Unexpected item in bagging areAAAARGGGHH here's 90p change in pennies

After 100 years, a key postulate of the third law of thermodynamics has been proven. We meet Lluis Masanes, one of the researchers responsible.

Not the new coin we want, but the new coin we need

How to make the most slices from just a few cuts of cake

Graphical linear algebra, slide rules and game theory in nature. Plus all your favourite fun pages in our spring 2017 issue.

We chat to the chief scientific advisor to the Home Office about the role of scientists and mathematicians in politics

Rediscover linear algebra by playing with circuit diagrams

Explain the strange dynamics of certain insects using game theory

Fermat's Last Theorem with complex powers, wrapped in a story every mathematician can relate to

When slide rules used to rule... find out why they still do

Factorisation is often used in cryptography. But there's something even simpler which turns out to be just as hard.

Folding origami, building networks, making projections and multiple dimensions!

What is the real story behind the lady with the lamp?

Mary Somerville fights against social mores to become one of the leading mathematicians of her time.

Win £100 of Maths Gear goodies by solving our famously fiendish crossnumber

Read more about the fire-breathing curves that appear on the cover of issue 05

Mathematical fashion advice for an ever-changing world

Prof. Dirichlet tackles political arguments and werewolves in answering your personal problems

Part 4 of our mathematical comic's adventure

Solve the puzzles that appeared in Issue 05.

How to model bread making!

Are you feeling ideal? In your prime? Discover your inner mathematical object (plus handy term explainer).

Make calculations easy with this simple-to-make slide rule

The definitive chart of the circle's greatest parts

Vote for your favourite geometry instrument

The answers to the puzzles that appeared in issue 05

How many did you spot?

Free launch party, 7.15pm. Come along! Free copies of Chalkdust, free pizza, and buy your own Chalkdust T-shirt.

What connects the products of twin primes with something you learnt in primary school and the number 8?

A tragic love story of shares and viral songs. To share, or not to share...

For those of you tackling this dilemma, here's your answer...

Some surprising mathematical facts

Here are some highlights of the first two years of Chalkdust!

Are you a winner?

Lindsay Lohan is really good at L'Hôpital's rule.

Why the infamous acronym needs revising.

Can you wear them and be taken mathematically seriously?

Before we say 'Goodbye' to 2016 let us highlight a few of the articles on the Chalkdust website.

It's the last day of the Chalkdust advent calendar, so there must be something very good behind today's door...

Behind today's door... a puzzle!

Behind today's door... A book review!

Behind today's door... More fascinating facts!

Behind today's door... Another carol!

Find your perfect partner with this wonderful tree diagram!

Behind today's door... A joke!

Agony uncle Professor Dirichlet answers your personal problems this Christmastime.

Behind today's door... A quiz!

This year's Chalkdust puzzle Christmas card

Santa's sack of scientific surprises

Behind today's door... a quiz!

Behind today's door... a puzzle!

This post was part of the Chalkdust 2016 Advent Calendar. Newton: “Are you going to the Fibonacci themed Christmas party?” Euler: “Yes! I heard it’s going to be as big as the two previous years put together.”

Behind today's door... a good joke!

Behind today's door... A binary magic card trick!

Winter is coming!

Behind today's door... The Chalkdust guide to Christmas presents

Behind today's door... a puzzle!

And on the fifth day, some rather Algebraic Golden Rings:

Behind today's door... a mathematical Christmas Carol

Santa's sack of scientific surprises

Behind the second door of the advent calendar, there is a puzzle

Get into the ChristMATHS spirit with the maths behind the popular Christmas carol!

How crime science, and the maths it uses, is helping the police fight crime

Here we have collected our favourite sets, from the Mandelbrot set to the Mahut-Isner set!

Using modern technology to understand geometry

The algebra will set your heart aflutter.

Constructing a spiderweb: in the spookiest and most horrific way possible!

The Great Fire of London, a little-known polymath and a Monument...

A blast from the past. Modelling battle grounds from ancient Greece.

Vote for your favourite part of a circle

Problem solving 101, proof by storytelling, plus the return of all your favourite fun pages in our autumn 2016 edition.

Never be stumped by a maths problem again, with this crash course from the ever-competent Stephen Muirhead

Follow our flowchart to find out where your true calling is

Part 3 of our mathematical comic's adventure

James Grime gets intimate with 'the most beautiful woman in the world' from the golden age of Hollywood

Colin Wright juggles Euler, doodling and Millennium problems

Don't be left behind... more mathematical fashion advice

Make your own treasures, guaranteed to be priceless on a future episode of Antiques Roadshow

Win £100 of maths goodies in our fiendish crossnumber

Solve the puzzles that appeared in Issue 04.

Pythagoras gave us so much more than a² + b² = c²

Exploring the connection between maths and art.

How to make mathematical crochet

The definitive chart of chalk's greatest colours

The geometry of psychedelic hallucinations

Can you solve it?

Warning: Contains SPOILERS!

Sit in your favourite chair and do away with those tedious algebraic proofs

Moonlighting agony uncle Prof. Dirichlet answers more of your personal problems

Diego Carranza tells you to stop worrying and dimensionally analyse the bomb

We chat to Andrea about crime, maths and her fluid career

Tuesday 11 October, 7.15pm. Come along! Featuring hundreds of free copies of Chalkdust, free pizza, and the chance to purchase a Chalkdust T-shirt.

A review of Timothy Revell's new book, describing the hidden mathematics behind our world

If only the Earth were flat...

Applying game theory to evolution

What can a 100-year-old result in topology say about weather and computers?

Donald Duck learns that there is “a lot more to mathematics than two-times-two”.

Exploring the maths on offer at this year's UK hacker festival.

How the queue size tells you when the next bus is coming

Believe it or not: Mathematics and Theology can coexist

Are you a winner?

Looking for a neat description of this useful matrix part-inverse

Were you a Choco Leibniz or a Fig Newton?

Can you solve these puzzles about differentiation and integration?

Difficulties in designing a voting system for referenda

Using Markov chains to calculate some interesting tennis stats!